The Exclusion Zone of Interaction Energy

When two particles interact owing to the interaction of their mutual electrostatic or gravitational fields, the fact that field energy density is proportional to field intensity squared means that there is a cross-product component of energy density separate from the self-energy components of either particle. This cross-product component is what is meant here by ‘interaction energy’. Our task now is to prove the following theorem:

Given two particles separated by a distance r and subject

to an inverse square of distance law of force, prove that

their interaction energy sums to zero within a sphere of

radius r centred on either charge.

Why is this important? It is important because in physics we face the problem of working out how fast that energy can transfer from its distribution over the whole of the field enveloping the particles to satisfy the Principle of Conservation of Energy by moving to or from the kinetic energy state which is seated with and shares the motion at the particle location.

For the electrostatic case, consider a charge Q in the figure above as developing a radial electric field V_Q at radius x. Imagine then a charge q distant r from Q developing a radial electric field V_q at the radius x from Q. Let y denote the distance from q and a point P under consideration at radius x from Q. Then, with φ as the angle between V_Q and V_q, we know that the interaction energy density component at P is:

(V_QV_q cosφ)/4π

© HAROLD ASPDEN, 2003

THE PHYSICS OF CREATION

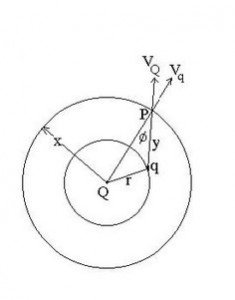
Fig. I.1

Also, V_Q is Q/x² and V_q is q/y². Now consider the volume of an elemental section of a spherical shell of thickness dx at the radius x, as subtended at P by a small solid angle from q. The elemental volume is y²/cosφ times this angle per unit thickness of the shell. If this is multiplied by the above expression after replacing V_Q and V_q by the above terms in x and y, we find that the cosφ term is eliminated as is the y²term, to leave us with an expression for the energy attributable to that elemental section dx of shell is Qq(dx)/4πx² times the solid angle mentioned. Since this does not depend upon y, we can evaluate the total energy component dE for the full solid angle of 4π to obtain:

dE = (Qq/x²)dx

Provided x is greater than r, the fields V_Q and V_q are in the same direction. With x less than r the two regions of the spherical shell intercepted by the same solid angle have opposite and cancelling interaction energies owing to the change of direction of V_Q relative to V_q. Thus within the radius r the interaction field energy sums to zero and so the proposition is proved.

 

Now we could have replaced Q and q by masses M and m and, by introducing G, the constant of gravitation, as a coefficient, reached exactly the same conclusion, namely that there is no net interaction energy within a sphere centred on M or, by the logic of symmetry, centred on m.

Accordingly, whether we have in mind the electrostatic interaction or the gravitational interaction, the energy transfer for change of separation distance involves energy having to traverse the distance r in going to or from the kinetic energy state in the process of transfer with field energy.

A separate mathematical analysis based on the use of MacClaurin’s Theorem can show that the interaction field energy distribution at radii beyond r is inversely proportional to the square of

x. From that one concludes that the precise distance energy travels in its transfer between field and kinetic energy is the distance r. The latter analysis is of record in chapter 1 of my book: ‘Physics Unified’ and also in a paper of mine published by the U.K. Institute of Physics [‘The Inverse Square Law of Force’, J. Phys. A: Math Gen., 13, 36493655 (1980)].

The importance of the theorem here presented is evident from the analysis in chapter (9) where we derived the equation (9.6) which led us to a physical foundation for the Neumann potential in terms of the Coulomb interaction. An equally important result, however, is that afforded by the clear analogy with the gravitational interaction, because equation (9.6) has a gravitational counterpart that is the basis of the point made in chapter 5 by reference to the expression (5.1). Physicists whose minds are entrenched in relativistic doctrine would do well to take stock of what has just been stated here, because one can see, from the argument that follows expression (5.1) in chapter 5, that the perihelion motion of planet Mercury can be explained by the simple classical logic of classical field theory, once the theorem presented in this Appendix I is given due attention.

© HAROLD ASPDEN, 2003