CHAPTER 2
Gravitation and the Continuum
Introduction
In chapter 1 our consideration of Earnshaw’s theorem established that all space must be permeated by a uniform continuum of electric charge. Since space overall is electrically neutral that continuum must contain numerous electrical charges having a charge polarity opposite to that of the continuum. Those charges can, notwithstanding Earnshaw’s theorem, form into a stable array, a simple cubic structure, which gives the aether certain characteristic properties.
One such property arises when there is an intruding presence of something that takes up space in that continuum. That something, if itself electrically neutral overall, may be assumed to be, in effect, the occupant of a hole in that continuum. Consider then two such holes, spaced apart, each of volume V within an electrical continuum of charge density σ. Given that the continuum charge, being everywhere of the same charge polarity, will repel itself owing to its electrostatic action, this means that those two holes will experience a force of mutual attraction.
It is tempting, therefore, to suggest that this may account for the force of gravitation should whatever it is that occupies those holes have the appropriate association with matter.
Note that the charges of the structured array, which will be referred to as lattice charges, will not interfere with this force of attraction because they merely attract the charge of the continuum, which, being of uniform charge density, takes precedence of position in keeping those holes away from these aether lattice charges.
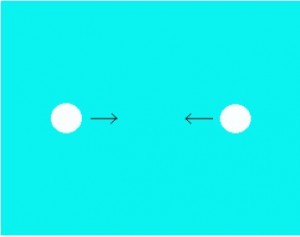
By way of illustration two such holes in a background charge continuum are depicted in Fig. 2.1. The arrows indicate a mutual force of attraction and one can imagine that as the holes come together, at the relatively slow speeds we associate with matter moving owing to gravitational attraction, the continuum charge will flow around the holes without there being any significant concentration of the charge densityσ.
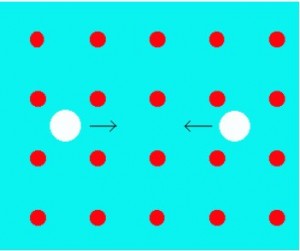
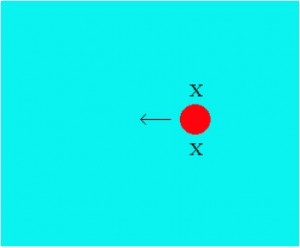
Fig. 2.2 depicts the presence of the lattice charges. The relative sizes of these compared with the gravitating holes are far from being represented by this figure. In fact, those aether lattice charges each have a volume that is quite enormous compared with the occupants of those holes, but even so, in displacing continuum charge they do not themselves contribute to the overall gravitational attraction between regions of space. The reason for this, as we shall see, is that the lattice charges are moving relative to the charge continuum at a very high speed, so fast in fact that, in being thereby forced by sudden pressure to flow around such a charge, the continuum charge is compacted in the regions denoted X in Fig. 2.3 to increase σ in those regions enough to ensure that the net continuum charge in the vicinity of the hole the lattice charge occupies compensates the effect of that hole and so does not contribute to the gravitational action. Gravitation is simply a question of the speed of whatever it is that takes up space within the charge continuum, a difficult concept perhaps, but one offering a convincing insight once we see how it leads us to the theoretical derivation of the value of G, the constant of gravitation. More will be said about this later, but meanwhile suffice it to say that we have introduced the theme of gravity as a property dependent upon the aether and our task now is to develop the formulation by which G is determined.
Fig. 2.1 Two such holes in a background charge continuum
Fig. 2.2 The presence of the lattice charges
Fig. 2.3 Continuum charge is compacted in the regions denoted X
Introducing the Graviton
Suppose that those holes of volume V are each associated with a mass M so that the mutual force of attraction between two such holes is (σV)2 at unit distance. The force law will be of inverse-square–of-distance form and so it can be said that this force is a gravitational force GM2.
If we know the value of σ and M has some standard value, which we can also evaluate in terms of the mass of the electron, then we can formulate that basic numerical factor involving G.
Now, the problem with gravitational mass is that it is not something that comes in specific units. The smallest amount of energy can exhibit the mass property. To cater for this under normal conditions Nature has adapted by creating two types of what we will here refer to as gravitons. These are unit charges e of either polarity that occupy those holes but they have different mass values.
Although it may appear to be mere assumption to say that Nature creates charged particles as needed and given the necessary energy, this is a fact evident from the phenomena of quantum-electrodynamics, where pairs of oppositely-charged electrons are produced by energy activity in the vacuum medium. These electron pairs, or rather electron-positron partnerships have a short lifetime, because, after their creation as charges spaced apart from one another, those charges come together by mutual attraction and are annihilated. They vanish to leave the energy quantum from which they were first created. Somehow the aether in its ongoing and well organized activity then contrives to recreate the electron and positron in a spaced relationship and so the cycle of charge pair creation and annihilation is repeated. The electron and positron are members of the lepton family. They are leptons and, indeed, those gravitons just mentioned are also leptons.
We need to know their mass values and the amount of space which their charges occupy if we are to derive a formula for the constant of gravitation G. We also need to know the value of the continuum charge density σ.
The latter quantity will be the unit of charge e as divided by the volume d3 of a cube of side dimension d, where d is the lattice spacing of the cubic array of those aether lattice particles that permeate the aether continuum. Later in this work it will be shown that d is 108πa, wherea is the radius of the Thomson electron. Therefore we have the following value for σ:
σ = e/(108πa)3 ……………………… (2.1)
Note that this equation applies without adjustment to cater for the volume of the lattice charges or particles of matter that might be present and sharing the motion of those lattice charges. The reason is that the compaction of σ in flowing around these intruding objects exactly balances the continuum charge displaced by their presence.
Concerning the charge volume to mass ratio of the graviton, this is complicated by the fact that there are two basic types of graviton, each type having a different role. By their creation and existence the gravitons create holes in the charge continuum which their charges fill. The ratio of the volume of those holes to the graviton mass is the primary factor determining G. Now, considering a group of three gravitons, if two have the same mass and so the same charge volume it is possible for them to exchange energy by very slight volume fluctuations where one expands in radius slightly as the other contracts slightly. Keep in mind that formula for the Thomson electron. As charge radius expands, so the energy and mass decreases and vice versa. The existence or non-existence of that group of three gravitons is a quantum transition for which gravitating mass changes in steps of whole units. Yet we need to cater for the smallest element of gravitating mass-energy. The third graviton in the group is deemed therefore to have the property that, if it sheds energy, its gravitational action, as represented by its increase in volume, will increase in just the right amount to match that quantity of mass-energy.
Now, a little exercise in mathematics will reveal that, if the graviton mass changes slightly, so the graviton charge volume will change in inverse proportion by an amount that is precisely three times the basic volume to mass ratio of that graviton.
Let the mass of one graviton form, the one of larger charge volume, be denoted as τ times that of the electron and the mass of the other graviton form be denoted as g times that of the electron, there being one g-graviton present for every two τ−gravitons. We will justify this ratio presently. What this then means is that, in terms of the charge volume to mass ratio of the electron, the graviton charge volume to mass ratio will be given by:
(2/τ3 + 1/g3)/(2τ + g) ………………….. (2.2)
Then, owing to that third graviton of a group of three, the g-graviton, having that threefold differential property in respect of the charge volume to mass ratio, we know that this ratio must equal:
3(1/g3)/g ……………………………… (2.3)
From which one can write:
3(τ/g)4 + (τ/g)3= 1 ……………………. (2.4)
and so find that:
g = (1.452627)τ ……………………. (2.5)
We can now progress in formulating the value of
G as:
G1/2 = (4π)(1/108π)3(1/g)4e/me …………… (2.6)
which provides the numerical factor concerning gravity that we set out to find.
However, we have yet to justify that 108π factor and we confront also the task of deriving the value of g from pure theory. Also there is that question of the two to one ratio of the τ- and g-graviton population, not to mention the many unanswered questions that can be raised as to how all this relates to the mass of many forms of matter that exist in our universe. We can but proceed in stages, but, by way of reassurance, the reader is invited to take note that the known varieties of charged leptons in physics are limited to but a few. There is the electron family, the heavy electron family otherwise known as the mu-mesons or muons, and then the even heavier lepton form, the tau lepton. It would seem that the latter is the τ−graviton. As to the g-graviton form this seems rather elusive in the spectrum of particle physics, but we shall point to some evidence later as we refer to the Japanese H quantum in chapter 9 [See section entitled: Numbers Game].
Meanwhile, from the above formulations (2.5) and (2.6), the reader may check the value that τ must have to satisfy the relationship between G as 6.67259(85)x10-8 dyne.cm2/gm2 and e/me as 0.527281x 1018 cm2 esu/gm. The answer you will find is that τ is 3485, meaning that the tau-lepton should have a mass energy of
1.781 GeV, some 3485 times 0.511 MeV, the mass-energy of the electron. On this basis g is 5062.3, which corresponds to a mass-energy of 2.587 GeV. Now, of course, these values for τ and g are empirical, having been derived from measured data on the assumption that the theoretical formulation is valid. However, it is our intention to show in chapter 4 that both τ and g can be derived theoretically and found to have values quite close, indeed very close, to those just presented and this will then mean that we have deciphered Nature’s message implicit in the value of the constant of gravitation G.
The 2:1 Graviton Ratio
The two to one ratio of the τ-graviton to g-graviton population can be justified in the following way. Imagine the τ-graviton as having the primary existence as a kind of parent from which the g-gravitons are born. The isolated τ-graviton is suddenly confronted with an influx of energy which it has to absorb. It has a unit charge e which can be positive or negative but we will take the case of it being positive. It has a certain charge volume. It can absorb energy by contracting in radius but we need to accept that space in the aether charge continuum is at a premium and, in contracting, some space becomes available for occupation by other charge. However, it takes time for the aether to adjust to changes associated with energy deployment.
The scenario envisaged therefore is one where the sudden influx of an appropriate quantum of energy absorbed by the τ-graviton will contract it approximately to the g graviton form, whereupon, to take up the volume of continuum vacated, two similar g-graviton forms will be created, one of charge +e and the other of charge -e. This is a process of lepton charge pair creation which must be followed soon thereafter by the onward quantum transitions that occur with a time delay as the movement of continuum charge imports the added space commensurate with the net amount of energy that is absorbed.
Since, for the case of the initial τ-graviton having a positive charge, the transition state has two positively charged pseudo g-gravitons and one negatively charged pseudo g-graviton, those quantum transitions, given that added space, will mean a decay back to the τ-graviton form with charge pair annihilation, except for the one case where the two positively charged g-gravitons decay before the third graviton in the group is affected. The residual three graviton group will comprise two positively charged τ-gravitons plus one negatively charged g-graviton. This is a combination which resists spontaneous decay by charge pair annihilation and so there is a physical basis for saying that the graviton system that pervades space will have two graviton forms, which exist in this two to one ratio.
As to the reference to the pseudo g-graviton form, this arises because, in dividing into three gravitons, the primary graviton will allot one third of its charge volume to each newly created pseudo-graviton with the result that the latter have a charge radius smaller by a factor of 1.44225 as compared with the τ-graviton. This means that during the rapid transition in adjusting to the energy fluctuations under consideration, the transient g-graviton form will be about 0.7% smaller in mass and so energy as compared with the ultimate g-graviton form. The completion of the transient phase therefore involves the residual g-graviton absorbing that extra energy.
It will, of course, be understood that it is the displaced volume of the continuum of charge density σ that matters in determining G, there being overall as many positively charged gravitons as negatively charged gravitons of either the τ or g form and graviton charge pairs being close enough together to preclude their actual charge from having any gravitational effect.
The Onward Quest
The task ahead involves us in an extensive analysis of the aether as that charge continuum permeated by those aether lattice particles. There is relative motion between these charge forms and that motion gives us the insight we need into the physical activity giving foundation for quantum mechanics and leads us to the derivation of equation (2.1) above and so that factor 108π.
Then there is the challenge of discovering how matter is created from the activity of the aether medium, and we will find that the creation of the proton and of those τ-gravitons, along with the g-gravitons, go hand-in-hand.
In this pursuit we find an answer to one of the great mysteries of physics. Physicists have long been puzzled as to why the muon, the mu-meson, the lepton particle form intermediate the electron and the taon, the tau particle, exists at all. It seems to serve no purpose whatsoever. Unlike the electron it is not seen as present in matter but yet it appears transiently in high energy particle physics.
It forms the subject of our next chapter but, as our story unfolds, you will see that the energy of a pair of muons is actually present in each unit cell of volume d3 of the aether. The resulting energy density is that pertaining to those aether lattice particles, meaning their charge volume as divided by their electric energy according to the Thomson charge formula.
We shall find, by the analysis from which that 108π factor is derived, that the aether lattice particle, which we name the quon, is of much larger charge volume than the electron, by a factor N, which will be shown to have the integer value 1843. This leads us to the equation:
Eo = (3/4π)(108π)3(1/N)4/3 mec2 …………… (2.7)
as the energy contained within each unit cell of the aether. With N as 1843, the factor in this equation has the numerical value 412.6658. Note that the muon that materializes in experiments of high energy particle physics has a mass somewhat greater than 206 times that of the electron. The numerical quantity just calculated represents the energy in electron terms of two virtual muons, meaning the lepton pair of muons that populate the aether.
The proton/electron mass ratio, P/me will, as we shall see, be that given by a quantity:
P/me = {9 – 2[(3/2)½ – 1]2 }Eo/2mec2 ………………….. (2.8)
which has the value 8.8989795 as multiplied by half of the above factor 412.6658, and so is 1836.152, which compares well with the measured value of 1836.152701(37).
This rather incredible degree of precision for the measured value of the proton/electron mass ratio is a daunting challenge for anyone who ventures in search of a theoretical explanation of this quantity. Having indicated that this theory, in its basic structure, succeeds to within a few parts in 10 million, it seems best now to await acceptance of the foundations on which the theory is constructed, namely the aether of the form introduced in this work, and leave onward progress for future generations of physicist.
One has to assume that the purpose of precision measurements of physical constants is to establish just how constant such quantities are, just in case they vary from place to place and with the passage of time. Also, whereas the constants themselves may not vary, history indicates that variation does occur in the assumed values, especially as new techniques of measurement are developed and more measurements are reported. However, it would seem that the proton/electron mass ratio as now measured is likely to survive as an adequate indication of its ultimate value.
Having introduced the τ-graviton and its alternative role as the tau-particle, the taon, it is appropriate here to note that the theory also gives a formula similar to (2.8) that accounts for its mass in terms of the rest mass of the electron. It is:
τ = 2(P/me)(1 – [(3/2)½ – 1]2)……………… (2.9)
which is 3487. This corresponds to a mass-energy 1.782 GeV. This is a little higher than the empirical value 3485 derived above from the G formula (2.6) and this raises the fascinating issue of what factors are at work in determining the quasi-stable energy state of the taon, a topic to be mentioned in the discussion chapter 9.
Finally, as part of this preliminary glimpse of the power of this theory in revealing how Nature determines the fundamental dimensionless constants of physics, it is noted that the quantity referred to by physicists as the fine-structure constant has also been deciphered as being that of the formulation:
hc/2πe2 = 108π(8/N)1/6 ………………. (2.10)
where N, as before, has that value 1843.
This expression is that of the inverse of the fine-structure constant which physical tables list has having a measured value of 137.0359895(61). In contrast our theoretical value as it applies in the true vacuum environment remote from matter is, as may be verified from (2.10), 137.0359153. In this case there are reasons why some slight upward modification of this quantity can occur for measurements made in laboratories that are moving through enveloping space at the speeds we associate with the cosmic motion of the solar system.
At this stage the author yields to temptation by pointing out that Einstein’s acclaim owes a great deal to the support he received from the Cambridge scientist Sir Arthur Eddington in the early years when his General Theory of Relativity was under scrutiny.
Eddington is well known also for his attempts to decipher Nature’s numerical factors, those dimensionless physical constants. However, at the time (1930) the fine structure constant had not been measured to a degree of precision which allowed one to be sure that that 137 figure was other than an integer. Eddington, who was impressed by Einstein’s four-dimensional notions of the space medium, evolved a theory by which 137 was seen as being:
(162 – 16)/2 + 16 + 1 which theory, in the words of B.W. Petley of the U.K. National Physical Laboratory (p. 161 in his book ‘The Fundamental Physical Constants and the Frontier of Measurement’, Adam Hilger Ltd. (1985), declared as coming:
“from considerations of the number of independent
elements in a symmetrical matrix in 16-dimensional
space where 16 equals 4 times 4 (4 being the number of
dimensions in Minkowski’s world).”
However, Petley then added the comment:
‘The theory lost respectability partly because Eddington
at first predicted the number as 136.’
It is noted that on that same page 161 of Petley’s book there appears a table listing theoretical expressions that have been, as the author puts it, ‘derived’ for the fine structure constant. The last entry in this table, in date sequence, before the experimental review value, is the one dated 1972, being the formulation of this author’s theory giving that value 137.035915, the reference being to the paper entitled: ‘Aether Theory and the Fine Structure Constant’ in Physics Letters 41A at p. 423. This paper was jointly authored, by this author, Dr. H. Aspden, who was with IBM at their Hursley Laboratory in England, and Dr. D. M. Eagles of the National Standards Laboratory, Sydney, Australia, who had contributed to the development of the theory by involving Dr. C. H. Burton who used the computer power of that laboratory to verify the author’s analysis of the electrical structure of the aether and thereby cooperating in the determination of the 1843 value of that factor N mentioned above.
However, reverting to the Eddington theme by reference to his book ‘New Pathways in Science’ (1935, Cambridge University Press) one surely must agree with a comment he made on p. 234 in introducing his theory:
‘I think that the opinion now widely prevails that the constants
(A), (B), (C), (D) are not arbitrary but will ultimately be found
to have a theoretical explanation.’
Here (A), (B), (C) and (D) were, respectively, the proton/electron mass ratio, the fine-structure constant, the ratio of the electrical force between an electron and proton to the gravitational force between them, and a rather curious quantity ‘the ratio of the natural radius of curvature of space-time to the wave-length of a mean Schrodinger wave’. Eddington, being Professor of Astronomy at Cambridge University, saw this latter quantity as important, its value, as he states, “depending upon the observed recession of the spiral nebulae and being about 1.2×1039.” Readers will therefore find it of interest, as we proceed, to see that this author’s theory can rise to the challenge posed by this fourth constant but we shall derive instead a formulation including the value of the Hubble constant as that is a more familiar quantity. See chapter 8.
It is somewhat hilarious to see that Eddington, in explaining his theory for the fine structure constant on p. 237 of that book, says the following:
“It is a feature of quantum theory that the particles are so much alike that we can never tell which is which; and we shall later see that this indistinguishability is actually the source of the energy that we are studying, so that we must not ignore it here. We have then to make one of 16 possible presents to one particle and one of 16 possibly similar presents to the other; but the particles are communists, not believing in private ownership, and it makes no difference which present has gone to which particle. There are 16 ways in which the commune can receive two like presents and 120 in which it can receive two unlike presents, making 136 in all.”
That is a curious way of saying that for each of 16 components to have two unlike or two like quanta, given that 16 of each variety are available, is, mathematically 16×15 divided by 2 plus 16.
However, Eddington was puzzled by the 136, when he really
needed a figure of 137. He ends his account on p. 237 by saying:
“Is it unreasonable to suggest that the fact that (each of those quanta) is one of a gang of 136 may have something to do with it? Apparently the majority of physicists think that it is. But for my own part the clue seems to me good enough to follow up.”
Clearly, Eddington is on the defensive here, but he struggles even further in seeking to derive a figure of 137. He concludes with the words:
“But, you may say, the fraction is really 1/137, not 1/136. I think if we can account for 136/137 of the quantum, the remaining 1/137 will not be long in turning up. There is a saying: One spoonful for each person and one for the pot.”
As to another of the basic constants, Eddington, by an argument based on wave functions, formulated a quadratic equation of the form:
10m2 – 136mmo + mo2 = 0 …………. (2.11)
relating two mass quantities and, taking mo as a standard unit, argued that, since the equation had two solutions for the value of m, these were, respectively, the electron mass and the proton mass. From this he derived the proton/electron mass ratio as having the value 1847.6, which, albeit in 1935, he declared “agrees very well with the observational determination of the mass-ratio”.
Eddington deemed there to be such a mass unit mo “furnished by the universe as a comparison object”. It would have a mass which the above equation shows as being 135.926 times the electron mass. Readers should note here that this author’s theory in no way supports the notion that a mass unit having this particular value exists and that we shall be using the symbol mo extensively later in this work to signify a different mass quantity, that of the aether lattice charges depicted in Fig. 2.2.
As to Eddington’s formulations, it was this kind of physics that caused the physics community to develop a great distaste for any attempts to account for physical phenomena that were guided solely by prior knowledge of the measured numerical factors involved. Where numbers seemed to dominate the argument this outlawed the theory and caused physicists to find more appeal in factors such as symmetry in mathematical formulations purporting to describe physical phenomena. Yet those numbers, as they evolved from high precision measurement, do convey Nature’s message, whereas the notional pictures of symmetry in an imaginary mathematical picture of space are merely the product of wishful thinking.
This author hopes, however, that with the passage of time since Eddington’s days and with the failure of existing techniques in physics to bridge the gaps which link gravitation with quantum theory and particle physics, physicists of this 21st century era will take note of what this author is offering in this work.
In our next chapter, we will come to the introduction of our overall theme, an account of the physics governing the creation of our universe, and this brings on stage the principal player, the virtual muon that was mentioned above as the primary energy form in our aether. In a sense, one could say that Eddington led the way in trying to decipher those numbers and he was headed in the right direction in postulating something in the universe having a standard mass intermediate the proton mass and the electron mass. However, it was too fanciful an argument to attribute those masses to the two solutions of a quadratic equation. The logical approach was to heed what J. J. Thomson had already presented as the mass-energy defined by the electron as a charge confined within a spherical volume of space and apply the general formula to other charges, including our unit mass form, the virtual muon, and combine these in an energy equation which seeks a minimum value.