Introduction

The mu-meson, or muon, is the ghost particle that inhabits all space. It is ‘ubiquitous’, which, according to dictionary definition, means it is ‘omnipresent; being everywhere or in an indefinite number of places at the same time’. Yet it has no recognized role in the structure of matter. When it does appear, as in cosmic radiation or as a decay product of the pi-meson, the pion, it has a fleeting existence, but it has been found to have properties of the kind we associate with electrons. Indeed, as mentioned earlier, it is sometimes referred to as ‘the heavy electron’ and it can, though only transiently, drive an electron out of its orbit around the nucleus of an atom and itself move around that nucleus, though in an orbit of much smaller radius.

So why do I refer to it as the ‘the ghost particle that inhabits all space’? Well, although it is there, everywhere in space, we cannot sense any resistance to our motion that we can attribute to such a presence. Why is that?

I can but suggest that it is because that is characteristic of its ghostly behaviour. You might, of course, be tempted to suggest much the same by assuming that I am merely ‘imagining ghosts that do not exist at all’, but do, please, stay with me as I show you how muons cooperate in the creation of the proton, the primary particle constituent of all matter.

Muons exist in pairs of electrical charges that can simply dissolve by annihilating one another and shedding energy which can meld into a uniformly dense background which allows those charges to reappear once an intruder has moved on. Indeed, we should not expect what is hidden from us in the quantum underworld of space to exhibit the same properties that are revealed to us by the atoms and molecules of our material world. If we meet resistance to our motion and press forward then we exert force and energy is dissipated as heat, but that heat energy is merely energy we have transferred to the obstructing object and that object is normally an atom, meaning a unit of matter and not a member of the lepton family that exists in the aether in a state of equilibrium consistent with uniform energy. If the medium that fills all space is already in a state of uniform equilibrium with pairs of muons conserving their energy in spite of material bodies moving amongst them and has already shed any surplus energy to create matter (protons and electrons), it will surely contrive to stay that way.

At best, therefore, with the exception of a phenomenon to be referred to as ‘vacuum spin’ or ‘aether spin’ as we proceed, we can expect that ghost world of the aether to cooperate in making it possible for matter in motion to suffer events in which that matter transforms itself into some other form of matter. In this respect, since electrons and positrons exist as matter and not as a constituent of the aether, we can look to the participation of the electron-positron lepton family when contemplating energy transfer processes that involve photons and deployment of kinetic energy as in electrodynamic actions. It would be foolish, indeed, to reject the picture of space as populated by the ‘ubiquitous muon’, solely on the ground that it offers no resistance to our passage through it. So, you may say, “If it is there and we cannot feel its presence, then why should we concern ourselves with its existence? Also, why use the word ‘muon’, given that is already the name of a particle that has materialised and has been seen as part of the particle spectrum in physics?”

The answer to this first question is that we can feel its presence indirectly, but not in the way one might expect. We do sense thosemuons by the existence of their reaction to our presence as a function of our mass, because they regroup in a stronger ghostly form (the tau-particle, the τ-graviton of chapter 2) in which they assume a role that we sense as the phenomenon of gravitation. In other words, they react to ensure that we mortals, for example, stay put on body Earth and do not drift off into outer space.

The answer to the second question is, to be frank with you, simply that I did not want to invent a new name for what I found was a pristine, newly born, version of an electrically charged energy quantum it is naked state, before it added just a little weight by consuming, as it were, an electron-positron pair, which then gave it a momentary presence as matter on the stage where we also perform. I have tried referring to it as the ‘virtual muon’ to distinguish it from the real muon, but, in the end, I have chosen to call it the ‘muon’ and, before leaving this chapter, I will enlighten you on the details of what I have just introduced.

Reverting to the first question and that link with gravitation, it is this gravitational connection that is the reason why we should not ignore the ‘ubiquitous muon’. The story of Creation is based on the muon as the building block from which the edifice of our universe has been constructed and the muon is also the agent giving birth to the action accounting for the force of gravity, without which the stars and planets could not have formed.

So please accept as our starting point that space is primarily a densely populated ocean of muons in which we, as intruding matter, have very little relevance. As we, with awe and due reverence, worship God, the Creator, those physicists amongst us might find a measure of satisfaction that can strengthen one’s faith concerning the Creation of the universe by studying what now follows from this introductory insight into the creative workings of Nature.

A snapshot overview of what lies ahead in later chapters is evident from Fig. 3.1.

Fig. 3.1

In view of the audacity of this attempt to explain Creation, it seems not inappropriate to use the following words to summarize, just briefly and only by this intervention, what is illustrated, in a biblical style of wording. The justification for this is that we now have 21^st century knowledge and the terminology of physics at our disposal but the sterile tone of modern scientific writings cannot convey the message of Creation with the same meaning as that of biblical times.

“And in the beginning there was Energy and that Energy

packaged itself into units of electrical charge, in pairs of

opposite polarity, that bear the name of Muons and which form a uniformly dense electrically-neutral plenum that fills all space. As this plenum evolved, the Muons gave birth to a system of Quons immersed in a uniform Continuum of electrical charge of opposite polarity to the charge of the Quons and there evolved from the chaos a state of order amongst those Quons as they acquired a rhythmic motion which was duly balanced dynamically by an accompaniment of Gravitons, specially created for that purpose. Then, with the passage of time, as some residual Energy needed accommodation, matter appeared in the form of Protons and Electrons which joined the Quons in the rhythm of their dance and which also had a Graviton counterpart. And those Gravitons, by their presence in the Continuum amongst those electrically charged Muons, gave rise to a force that acted over vast domains of space and between all matter in each such domain, thereby drawing that matter together to form a star or pair of stars in each of those domains. And so it was that the universe was created and those Protons and Electrons combined to form atoms some of which developed into the more complex forms from which Mankind and other life forms duly evolved.”

With this introduction the reader now has a choice. That muon form we see in experiments on particle physics is found to have a mass-energy by which a pair of muons would have a combined energy of 211 MeV. The reader can move on immediately to Chapter 4, to be introduced to the creation of the proton from those muons and, hopefully duly impressed by what has been discovered. Nature’s coded message there resolved is the one encoded in the number 1836.152, the proton/electron mass ratio.

However, if the reader is reluctant to be led along a path where numbers may seem to be more important than the underlying physics, then such a reader may prefer to plough through the remainder of this chapter, where we will delve into some of the intricacies that distinguish the muon in its primeval, bare or ‘virtual’, form from its real form in its transient presence as matter. Numbers will still play a major part in this pursuit, simply because the measure of muon lifetime in seconds is a mere number, as is its mass ratio relative to the electron, but at least you will see that number derived from a rigorous theoretical exercise in physics. The reader needs to keep in mind that so much of what one reads in advanced theoretical physics is dominated by complex notions and even more complex equations which really have no clear meaning and, if given numerical form, seldom conform in precision with what is actually measured. It is very different from the realm of the engineer where one can design machines and structures that, in operation, match up well with theoretical prediction. I will give one example of this that pertains to the muon at the outset as we now proceed, but do realise that, in addressing this muon issue in detail, I am departing along a kind of side-track from the line of argument that I would otherwise follow, given a reader interested to learn something new by following a new theoretical route without being handicapped, in a sense, by prior indoctrination, as, for example, on the subject of time-dilation. You see, the muon and its lifetime variation as a function of speed is deemed to be a test verifying Einstein’s Theory of Relativity and certain aspects of that theory are not in keeping with the process of Creation that I have introduced above.

I hope these words of introduction will justify the diversion now offered by the remainder of this chapter.

The Muon Lifetime

The muon, as reported in Review of Modern Physics, (v. 48, 2, Part II, April, 1976), has an observed mean lifetime of:

2.197134 +/- 0.000077 microsconds and it is an interesting task in physics to discover what it is that determines this particular lifetime period.

Physicists have seen a way in which to progress in this quest by using the techniques of what is termed ‘Electroweak Theory’. Under a chapter heading ‘Feynman Rules for Electroweak Theory’ on p. 236, Bailin and Love in their book ‘Introduction to Gauge Field Theory’(Adam Hilger Ltd, 1986) derive a theoretical value for the muon lifetime, namely:

2.90 +/- 2.61 microseconds

Of this result they say: “Thus, while our result is consistent with the data, the large errors on this theoretical value of muon lifetime mean that this is hardly a rigorous test of the theory.”

So if one is really interested in delving into the theoretical foundations which govern much of particle physics and particularly physics pertaining to leptons, the realm of quantum electrodynamics, one presumably should not be concerned with the kind of aether advocated in this work.

However, do note that the theoretical derivation of numerical quantities that arise in basic physics is seen as a test of the theory involved. So let us put our alternative theory to the test by trying to derive the lifetime of the muon.

First of all, we ask, “What is a muon, meaning the one that appears in the matter frame of high energy particle physics?” It will, according to the theory already presented, comply with the formulation used by J. J. Thomson for the electron, though having its charge radius reduced by a factor equal to the muon/electron mass ratio. Yet, given that electrons and positrons are prevalent in the quantum electrodynamic arena, one can wonder if a simple positive muon charge might attract two electrons and form a three-charge entity having the character we recognize as a negative muon.

With this in mind, the author has surmised that such muon entities might well comprise a core charge that, of itself, has a mass that is an odd integer multiple of the electron mass, that multiple being

207. The reason is that those two electrons, in repelling one another, take up positions in near-contact with the muon charge but at diametrically opposite locations and, by adding two units of electron mass as offset by the negative mass-energy of their electrostatic interaction, the net result is that the muon entity would have a mass of approximately 206.75 electron mass units.

The measured muon/electron mass ratio is 206.76835(11) (Physical Review D, 25, 652; 1982) but onward analysis which involved a resonant wave interaction governing the actual spacing of the core muon body and the satellite electrons (or positrons) led to the author’s theory giving the mass ratio as:

207 + 2 – 9[207/(208+2π/9)]/4 which is 206.7683078. The full theory for this is of published record in the Italian Institute of Physics publication Lettere al Nuovo Cimento (38, 342; 1983). So, this result being precisely in accord with the measured value, you see why the author is confident that this model of the muon is the proper basis from which to seek to explain the lifetime and so test the theory further. Indeed, what is now to be described supercedes the theory for that lifetime that features at p. 146 of the author’s book ‘Physics Unified’, published in 1980.

Concerning muon lifetime, physicists well know that Einstein’s theory requires the lifetime of a particle to increase with speed according to a relativistic formula, just as the same theory requires the energy of a particle to increase according to the same formula. Already in this work, in discussing electron energy theory as advocated by J. J. Thomson, we have seen why the mass of a particle moving at high speed is increased in accordance with what is observed, as in the Kaufmann’s experiments of the 19^th century. There is no particular merit in Einstein’s derivation of the mass-increase formula. Concerning muon lifetime, however, the lifetime enhancement with speed is best explained once one has an acceptable theory for the muon lifetime with the muon virtually at rest. Otherwise, it makes no sense at all to theorize about the lifetime changing with speed when it would seem that all one needs to do is to argue that lifetime is proportional to mass-energy and, as Einstein does, then argue that time itself is perceived to change in its rhythm as a function of speed.

We will confine our concerns here with deriving that muon lifetime for the rest condition, but if readers wish to see how this same aether theory does give account of muon lifetime dilation with speed then the appropriate reference is this author’s paper entitled: ‘Meson Lifetime Dilation as a Test of Special Relativity’, (Lettere al Nuovo Cimento, 38, 206; 1983). Einstein’s theory is not involved in this exercise, but one can point to a section of that paper where one may read:

“A primary publication on this subject is that of Bailey and Picasso (Progress in Nuclear Physics, 12, 62; 1970) who measured muon lifetime at very high speed for which the theoretical relativistic value was 26.69 microseconds compared with a rest lifetime of 2.198 microseconds. Thus … (this author’s theory) …. tells us that the observed lifetime at this value (of energy at the high speed compared with rest-mass energy) should be 0.56% low compared with the relativistic value. This is quite small, but it is also significant because Bailey and Picasso reported measurements to an experimental accuracy of 0.2% and did in fact find that the observed value was lower than the relativistic value by 1.2%. Though they regard this as adequate agreement the difference was sufficiently concerning for them to speculate at some length about the possible reasons for the difference. Only further research can verify the speculation, but it can be said that the question remains

open and the indications are that the relativistic

expectation is up to 1% above the measured value of the

muon lifetime at these energy levels.”

Concerning the muon and this author’s theory, one further point of interest before we come to the derivation of that lifetime property is that the very same model which the author used for deriving the mass of the muon gave a full account of the muon g-factor, again without involving Einstein’s theory or, indeed, the standard arguments based on Feynman diagrams. The principles involved are much the same as those this author has applied in deriving the anomalous magnetic moment (g-factor) of the electron, the latter being presented in this work as Appendix III.

The periodical reference is this author’s paper: ‘The Muon g-factor by Cavity Resonance Theory’, Lettere al Nuovo Cimento, 39, 271; 1984. The theoretical value for the muon g-factor was found to be 2(1.001165918), a result in quite remarkable agreement with the then measured value of 2(1.0011659230), given the simplicity of the theory used.

One can see, therefore, in this digression from the main topic of this work, that concerning the physics of Creation, we need not lose sight of the reality of what is observed concerning the muon in our laboratory experiments. This point is made particularly because, in introducing the ‘ubiquitous muon’ of the aether medium as something different but active in the quantum underworld of space, it may seem that we are invoking arbitrary assumptions, whereas the analysis, once we reach the realm of quantum theory, will be seen to lead us to a mass-energy for that ‘ubiquitous muon’ which is indeed very close to that of the real muon which reveals itself in our experiments.

Now we come to the question of the muon lifetime. The aether we are considering in this work has a unit cell volume that is (108π)³ times the cube of the radius of the electron charge and it will emerge as we proceed that the aether has a rhythmic cycle at the Compton electron frequency, the photon frequency corresponding to the electron mass-energy quantum. In every such cycle there is a quantum-electrodynamic event associated with the energy quantum of a pair of virtual muons but such events occur at random positions in space. The proposition now advanced is that if those two electron charges associated with the core muon form sustain a hit simultaneously in the same rhythmic period, then the muon will be conditioned for decay.

By ‘hit’ it is meant that the virtual muon of opposite charge polarity is created within the space occupied by the electron charge. By ‘conditioned for decay’ is meant the transient creation of a system which statistically has the prospect of decaying in the manner now explained.

Given one unit of muon energy plus two similar units owing to the impact of the two virtual muons, there are three units of muon energy. Given also the fact, as we shall see later in this work, that the space occupied by material charge forms is conserved in particle reactions, the muon, when subjected to such a ‘hit’ is converted to a higher energy level, pending decay or reversion to the normal state.

Some muons so affected are transiently elevated to an energy level that is less than three times their normal rest-mass energy, whereas others are elevated to an energy level that is greater than three times their normal rest-mass energy. The latter are the ones that experience decay. The energy deployment determines the ratio of the two states as being 17:8, meaning that the chance of decay for each ‘hit’ is 8 in 25.

This immediately leads us to the formula for muon lifetime as being: (25/8)(3/4π)²(108π)⁶(8.093)10^-21 s which is:

2.199 microseconds a value that is within one part per thousand of the experimental value.

If a muon core charge +e is suddenly forced to shed its two electron (or positron) associates and is driven to an energy level some three times greater then one could contemplate the space occupied by its basic charge form being shared equally by three charges, +e, -e and +e. Each such new charge form will have a charge radius that decreases by a factor equal to the cube root of 3. Conversely, each such charge will have a mass-energy enhanced by the same factor 1.44225, according to the Thomson formula already introduced.

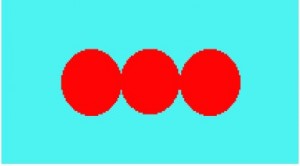
Now, depending upon how these three charges arrange themselves in a group, the overall mass-energy can be greater or less than that of three basic muons.

Fig. 3.2

One possible configuration of the three charges is depicted in Fig. 3.2 and such a charge group has an electric energy that, in the basic muon units, is 3 as offset by the sum of three components of electrostatic interaction energy. This leads to the following quantity:

3 – 0.75 – 0.75 + 0.375 = 1.875 and, upon multiplication by 1.44225, this becomes 2.7042.

We could then expect that, since muons are involved and are subject to pair creation and pair annihilation, the number of such configurations that might be created as a group would require an energy threshold that is an appropriate integer multiple of the basic muon energy quantum. If now one multiplies 2.7042 by successive integers until one comes to a value that is itself well within one part in 1,000 of being an integer value, then it is found that the necessary multiple is 17.

As such a group forms by deploying a total of three muon units of energy at each step there will be a surplus of energy of 0.2958 units, some of which is likely to be deployed in helping to create a more energetic version of that three-charge system, as shown in Fig. 3.3.

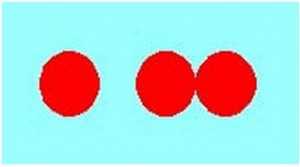
Fig. 3.3

Here one of the charges is separated from the other two and the energy at separation to a distance far in excess of the charge radius may then be as high as:

3 – 0.75 = 2.25 times 1.44225, which is 3.2451.

It seems then possible that, as each of the Fig. 3.2 charge combinations forms by shedding that energy of 0.2958 units, there is the possibility that the two such quanta of energy shed by the nearby activity of creating two similar charge combinations will converge on the primary combination and act to separate the charges and so form the Fig. 3.3 charge system. This is because 2(0.2958) exceeds (0.2958

+ 0.2451), meaning that 0.0507 units of energy disperse at this second stage of action to leave three charge combinations, two of the Fig. 3.2

form and one of the Fig. 3.3 form. Therefore, in progressing to the threshold stage at which 17 of the Fig. 3.2 combinations have been created one has by then also created 8 of the Fig. 3.3 form. This is very satisfactory because 8 times 3.2451 is 25.96, which is itself within one part in 650 of being an integer.

As to the muon decay process, the Fig. 3.2 charge combination will revert to the basic muon state because two adjacent charges will annihilate one another and, being close to the remaining charge, their charge volume will be conserved for use by that residual charge as it sheds surplus energy. However, for the Fig. 3.3 charge system, the decay by charge pair annihilation occurs at a distance from the residual charge and that charge, in shedding energy, cannot capture the space vacated by the charge pair and so revert to its basic muon form. It stands in isolation and lacks the stability assured by the close presence of other charges having the same properties. The latter is important because a group of three or more like charges conforming with the J. J. Thomson formula for energy in terms of charge volume can preserve equilibrium by exchanging energy and still conserve their overall energy and charge volume. Hence, owing to that isolation, the Fig. 3.3 charge combinations must decay at the end of the rhythmic quantum cycle.

For this reason there is the statistical probability that in every group of 25 muons that happen to be excited to the potentially-unstable condition, there are 8 that will decay and 17 that survive decay, thereby substantiating the above formula for the muon lifetime.

Now, the author will be the first to concede that the above account is somewhat complicated in the derivation of that 8 to 17 ratio and there may be a better alternative yet to be discovered, but it does give what seems to be the right answer. Accordingly, it is included here for the record and also to make the case that, in comparing this aether theory with Einstein’s theory or what is referred to as ‘Electroweak’ theory, there is really no contest, given that Einstein’s theory does not even offer any estimate of the muon lifetime that one can define in numerical terms, whereas the other standard theory gives, as stated above, a figure well off target and is anyway subject to an uncertainty having a standard deviation amounting to 90% of the estimated value.

Concerning other particle lifetimes we shall not, elsewhere in this work, be seeking to derive further theoretical values as evidence supporting this theory. Our primary concern in this work is the problem of Creation on the universal scale. However, by way of reference, readers interested in particle lifetime theory may find it of interest to look up the following papers by this author.

‘A Theory of Pion Lifetime’, Lettere Nuovo Cimento, 33, 237 (1982).

‘A Theory of Neutron Lifetime’, Lettere al Nuovo Cimento, 31, 383 (1981).

‘The Finite Lifetime of the Electron’, Speculations in Science and Technology, 7, 3 (1984).

The latter paper discusses the reason why the electron has a finite lifetime evident from its electron tunnelling properties. It must have a lifetime if the above theory for muon lifetime is correct, because it is the dual ‘hit’ of two electrons (or positrons) as a target that gives basis for muon decay. That electron lifetime, based of course on a single ‘hit’, is of the order of 0.75×10^-13 seconds and may be derived from what has been presented above by using the formula:

(3/4π)(108π)³(8.093)10^-21 s

One of the most fascinating questions one can then consider is whether the proton has a finite lifetime. As for the electron, standard physics offers no suggestion that these two most fundamental of all particles of matter may be subject to eventual decay. However, may not the reason for this be that decay sheds energy which our aether has to take under its wing and look after pending finding a new home for that energy in the system of matter? It is simply a question of equilibrium as between the energy the aether can store to meet its own structural needs and surplus energy it may possess transiently pending shedding it to create matter. Given then that the basic forms of matter in the local space domain of our experience consist in negative electric charges assuming the form of the electron and positive electric charges forming as protons, need we be surprised if we were to find that both an electron and a proton may have finite lifetimes but, upon decay, they are recreated almost at the point where they suffered their demise?

As to the proton, though we shall see in the next chapter how it is created, we will later in this work discuss an aspect of this process that explores the broader picture of proton creation even in outer space, or rather the aether’s attempts at proton creation that fail owing to lack of surplus energy. That ongoing scenario of an aether subject to quantum fluctuations involving those ‘ubiquitous’ muons in trying to create protons is one from which we can derive the theoretical value of the Hubble constant as a feature of a non-expanding universe necessarily set in that aether. Such is the scale and scope of the subject of this book concerning Creation.